3:4

When we look, we count
7 = 3 + 4
3:4 in a building composition
Proportional Continuity
The Plastic Number and the Golden Section

I When we look, we count

For Dom Hans Van der Laan, order is the underlying principle of everything. Nature, as God’s creation, is unfathomable. Its forms have countless sizes. Architecture, as the fundamental HABITUS is there to surround us like clothing and to make our environment readable. As such, it is closely linked to our process of knowing. Van der Laan defines ‘to inhabit’ as being able to enter into a relationship with a space, being able to measure that space.

This is the EXPRESSIVENESS of architecture.

When we measure, we round off to whole numbers and we count. We intuitively estimate the size of something by choosing a yardstick in space, using it as a foundation for counting that space.

II 7 = 3 + 4

There are two ways of READING space: COUNTING and MEASURING. When confronted with two measures of the same size, one COUNTS. In the case of unequal parts, one MEASURES or compares. In order for space to be clearly readable, architecture makes measuring as straightforward as counting.

To enable this, Van der Laan asks himself the question: what is the minimum difference between two sizes, so that we can differentiate them clearly, in other words, so that we can count or name the difference?

The answer is 3:4.

So Van der Laan does not start from 10 and divides this in equal parts, he starts from 7 and divides this in 3 and 4. This opens up a whole new world of designing through relations and compositions beyond mirror symmetry.

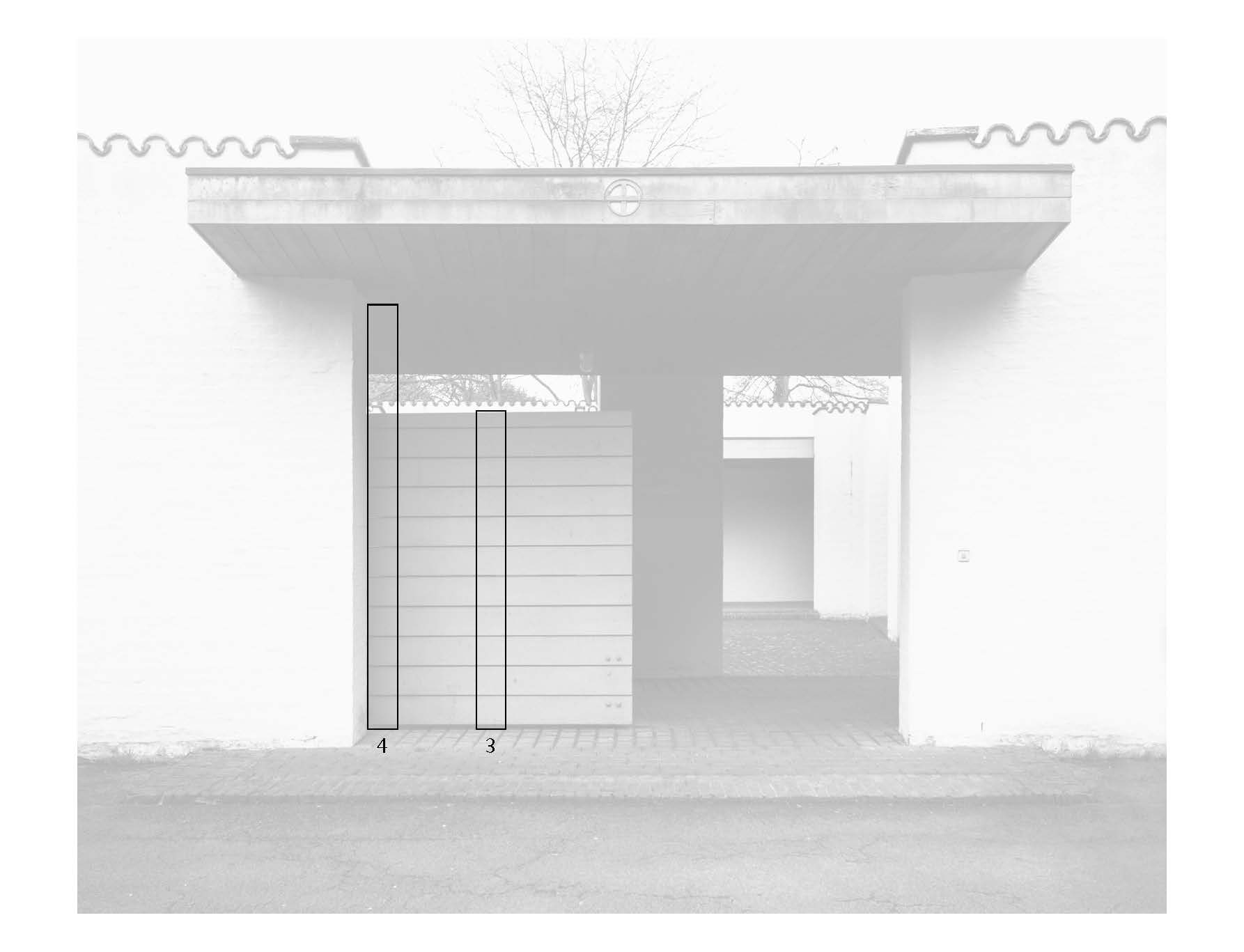
7 is divided in 3 and 4.

The difference between 3 and 4 is the minimum difference between two measures, so that one can compare them and name the difference clearly.

The necessary margin between two sizes is 1:4.

Van der Laan named this the PLASTIC NUMBER or GROUND RATIO. 3:4 is an approximation of 1,324718…

Mirror symmetry: in the West we are used to design like this: subdivide a length in equal parts.

This is a static way of approaching design. There is no rhythm or hierarchy, no dynamism or tension. Only counting.

III 3:4 in a building composition

In Dom van der Laan’s buildings, everything is interrelated through 3:4.

‘This is what the Ancients called symmetry; not in the sense of two identical halves, but in the sense of the proportion between the sizes of the parts of a building, from the smallest to the whole.’ (VDL, AS IX 6)

A beautiful example of these 3:4 compositions is the entrance of Roosenberg Abbey.

The entrance of Roosenberg Abbey is not a clear opening in a wall. It is a superposition of several building elements, rendering it into a layered composition, full of tention and ambiguity.

Through the lowering of the canopy, it becomes autonomous from the wall. As such, the opening presents itself as a slit between two walls. Between the canopy and the small step on the ground, a space arises.

Walking underneath, through, passing by, … are all laid out in different moments in time.

The wooden gate is lower, in a symmetrical ratio of 3:4 to the opening. It is positioned behind the wall opening. The thickness of the wall is exposed in all its robustness, while the wooden gate seems to be of another order, invisible when opened.

Symmetry: two lengths of different forms are compared.

III

The opening of the entre has the eurythmic proportion of 3:4.

Eurythmy: width and height within one form are compared.

Roosenberg shows no mirror symmetry. The first thing encountered upon entering the forecourt is a column, placed in an unconventional way that blocks a logical entry. It is placed there with a purpose, inviting one to slow down. This column is not positioned in the middle of the slit, but shifted within the opening by a ratio of 3:4.

The careful composition of the entrance space already testifies to the system applied throughout the whole complex, a succession of interlocking spaces between outside and inside. Every building element is there to invite one to move consciously through the space, to enhance awareness, drawing attention to the here and now.

IV Proportional Continuity

The Plastic Number 1,325… has a continuous growth pattern. This growth occurs three-dimensionally.

Moreover: the Plastic Number represents the smallest proportional growth of three-dimensional objects.

1 + a = a³

An elementary block has three sides which relate as 1:1,325…

The smallest side is 1.

The middle side is a or 1,325…

The largest side is a².

The elementary block can grow continuously using the Plastic Number a.

When enlarging the elementary block into a side a³, we notice that:

1 + a = a³

This is different than the golden section , which defines the Fibonacci series, and is defined as 1 + a = a².

V The Plastic Number and the Golden Section

With the Plastic Number, Dom Van der Laan found a proportion that allowed all six segments produced by two subdivisions to be a continuous ratio, forming an additive geometric progression.

The discovery of the Plastic Number grew out of a dissatisfaction with the Golden Section. Dom van der Laan claimed that this proportion did not allow for a certain harmonious progressive subdivision of a measure. When subdividing the largest measure according to the Golden Section ratio, one ends up with two equal parts. This is the first definition of the Plastic Number, as explained by Dom Van der Laan in his first lecture series in Leiden, on 16 January 1943.

Subdivision of AB into AC and CB according to the Golden Section:
1:1,61803…

Subdivision of the largest part CB into CD and DB according to the Golden Section.
The result is two equal parts, AC and CD.
There is no continuous subdivision.

AB : BC = BC : AC
AC = CD

two equal parts
‘sameness’

Golden Section: no continuous series
Mathematical definition: 1 + x = x²

Subdivision of AB into AC and CB according to the Plastic Number:
1:1,324718… or ca. 3:4

Subdivision of the largest part CB into CD and DB according to the Plastic Number.
C:D and A:C relate as 3:4.
There is a continuous subdivision.

AB : AD = AD : BC
= BC : AC
= AC : CD
= CD : BD

Plastic Number: a continuous series of six parts interrelated by ca. 3:4
Mathematical definition: 1 + x = x³

Read more about ‘A 3 dimensional tower on a 2 dimensional façade’

Practice A Bell Tower in The Netherlands Design Analysis A 3 dimensional tower on a 2 dimensional façade